Main Content

Lesson 2: Forward Contracts I Part 2

Forward - Pricing and Valuation

Forward Contract on Investment Assets with Known Dollar Income

Note: If you need further assistance in understanding this concept, view the Forward Contract on Asset with One-time Known Income video by clicking on the Instructional Videos link in the left menu.

The Issue: If the underlying asset of a forward contract has income before the maturity of the forward contract, a synthetic forward method requires adjustments with the income. The table below compares cash flows between the forward (top half) and the synthetic forward (bottom half).

Table 2.2. Comparison of Cash Flows of Forward and Synthetic Forward Methods
Forwardt = 0t = 3 mot = 9 mo
------- F(0,9)
------+ one share of stock
Synthetic Forwardt = 0t = 3 mot = 9 mo
Borrow+S0---FV9(S0)
Buy one share-S0--+ one share
Dividend--D3mo+FV9(D3mo)

The equality of the cash flows requires:

Equation 2.1. - F(0,9) = - FV9(S0) + FV9(D3mo)

Since

FV9(D3mo) = PV0(D3mo) · (1+r9mo)9/12

and

FV9(S0) = S0 · (1 + r9mo)9/12

⇒ Equation 2.1. yields:

F(0,9) = FV9(S0) - FV9(D3mo) = {S0 - PV0(D3mo)} · (1 + r9mo)9/12

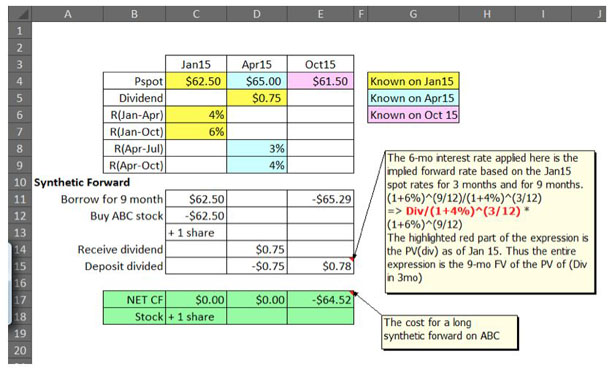
Example 2.6. (Forward Price on Assets with Known Income):

On January 15th, ABC stock is priced at $62.50 and will pay a $0.75 dividend in April 15th. The 3-month interest rate is 4%, the 9-month interest rate 6%, and the 12-month rate 7%.

  1. What would be an equilibrium price of a forward contract on the stock expiring on October 15th?
  2. What would be the value of a long forward contract above on April 15th, ex dividend? The stock price is $65.00, and the 3-month interest rate 3%, the 6-month rate 4%, and 9-month rate 5%.
  3. On October 15th, the expiration date, the stock price is $61.50. What is the value of the forward contract?

Click on Example 2.6. Solution to view the solution.

Example 2.6. Solution:

STEPS - using the discrete compounding method:

  1. Consider a synthetic forward: Buy ABC stock at the spot price with borrowed money for 9 months:

    On January 15:

    CF1/15 = + 62.50 (loan) – 62.50 (pay for stock) = 0
    + 1 share of ABC stock
    + a forward contract to deposit $0.75 on 4/15 for 6 months
    [implied forward rate (1 + r9-mo)9/12/(1 + r3-mo)3/12]

    On April 15:

    + $0.75 dividend
    - $0.75 [deposit dividend at (1 + r9-mo)9/12/(1 + r3-mo)3/12 ]
    CF4/15 = 0

    On October 15

    CF10/15 = - 62.50 · (1 + r9-mo)9/12(← loan repayment)
    + 0.75 · (1 + r9 - mo)9/12/(1 + r3 - mo)3/12 (← FV of div)

    ⇒ Since the cash flows on January 15 & April 15 are both ZERO, the forward price should be equal to the cash flow on October 15 from the synthetic forward, CF10/15:

    F(Jan 15, Oct 15) = FV10/15(S1/15 - PV1/15(Div4/15))
    = (1+6%)(9/12){62.50 - 0.75/(1+4%)(3/12)} = $64.52

  1. The value of the long forward contract on April 15th is:

    (Spot Price on Apr 15) minus PV of F(Jan 15, Oct 15) on Apr 15
    = 65 - 64.52/(1 + 4%)(6/12) = 65 - 63.27 = 1.73

  1. The value the long forward contract on October 15th is:

    (Spot Price on Oct 15) minus PV of F(Jan 15, Oct 15) on Oct 15
    = 61.50 - 64.52 = - 3.02

The figure below is an Excel Worksheet solving this problem:

Figure 2.6. Example 2.6. Solution Excel File
 

 


Top of page