Lesson 2 Commentary
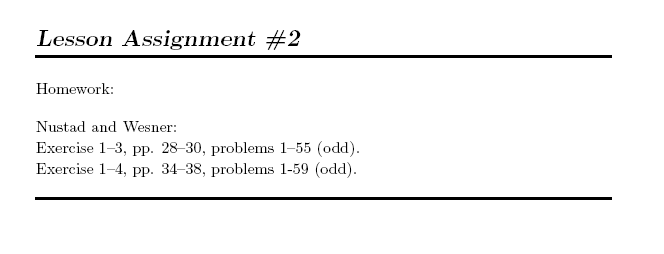
The real numbers are an example of a mathematical structure known as a field.Because of this, the real numbers have the following properties governing addition and multiplication:


Reading Assignment: Nustad and Wesner: Sections 1–3 and 1–4, pp. 24–28 and pp. 31–34.
Learning Objectives
After completing this lesson, you will be able to:
• recognize the properties of real numbers, by definition and in examples
• understand the addition and multiplication properties of equality, the zero
factor property and the double-negative property
• identify the terms of an algebraic expression and the coefficients of each term
• recognize like terms in an algebraic expression and employ the distributive
property to combine those like terms
• use the distributive property to remove grouping symbols in an algebraic
expression
• apply the correct order when simplifying expressions containing more then
one arithmetic operation


In order to illustrate why the distributive property of multiplication over addition
is so important in the study of algebra, algebraic expressions and their component
parts must first be defined.
Algebra allows for operations to be performed when not every part is known. An
example of this might be the sum x + 3, or the product 5x. In each of these, x
represents (or holds the place) of the unknown quantity. Since this value changes
depending on the statement of the problem, the x is called a variable. The numbers
in each expression, in one case a 3 and in the other a 5, are referred to as constants,
since their value never changes.
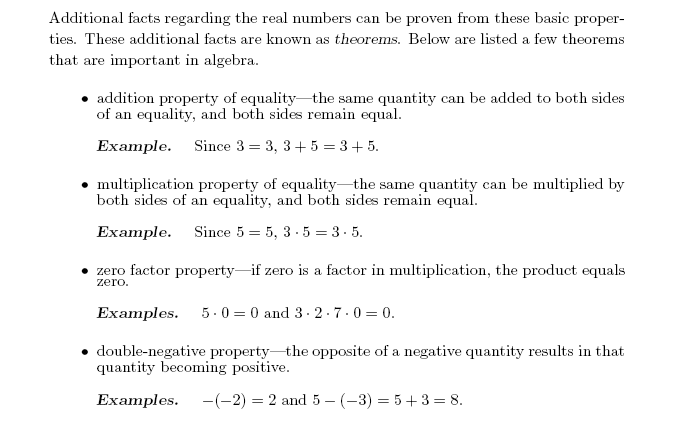
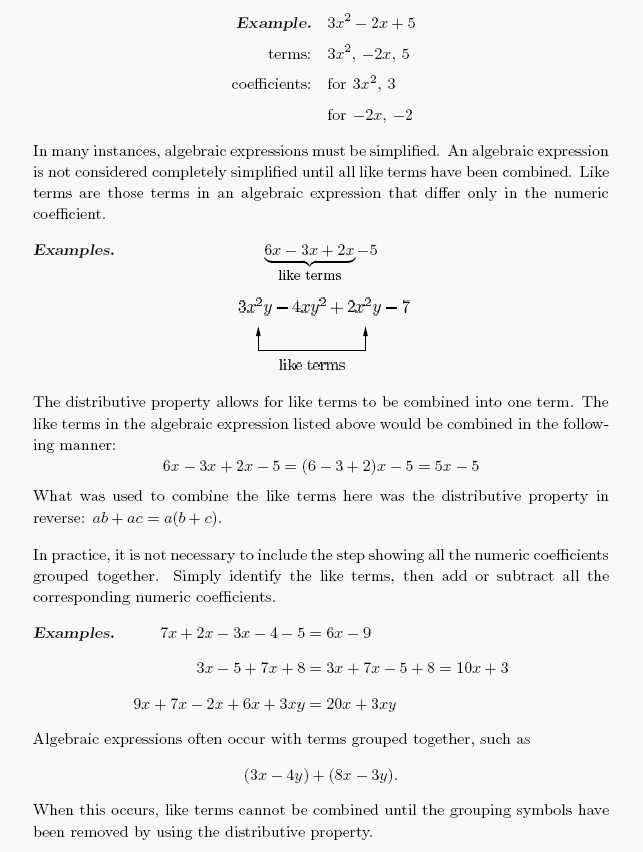
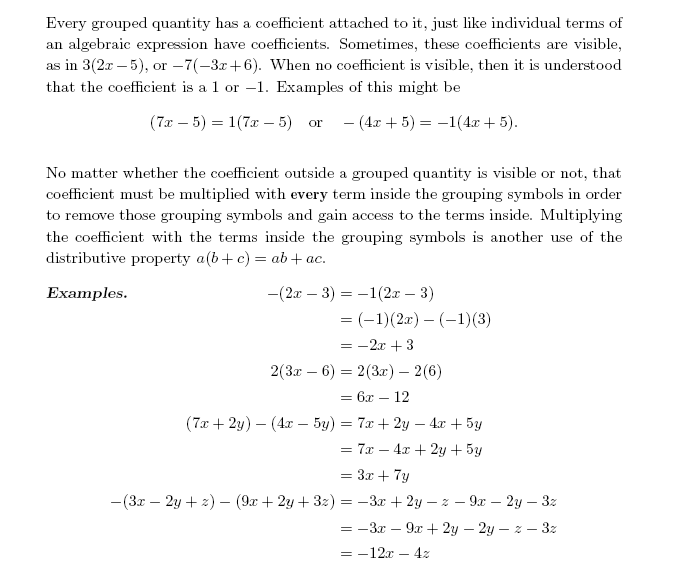
Much of the work in algebra involves algebraic expressions. These expressions consist of one or more variables and constants. The parts of an algebraic expression are called the terms. Each term is separated by an addition or subtraction sign. Terms are made up of a constant, and usually one or more variables. All parts of an individual term are multiplied together. The quantity multiplied by a given variable in a term is known as the term’s coefficient. In most cases, the coefficient is the constant portion of a term. If a term does not have a visible coefficient it is understood to be a 1. Note that the sign that precedes the term is part of the term.
Many times there are multiple arithmetic operations occurring in one problem, algebraic expression or formula. One standard for prioritizing the order in which these operations are performed is needed, as solving using different orders can resul tin different answers. Consider the following:
INSERT CAMTASIA MOVIE WITH EXAMPLES of the following material
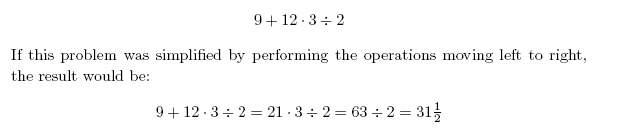

1. Perform any operations found in grouping symbols, absolute value signs, or in
the numerator or the denominator of a fraction.
2. Simplify any exponential expressions.
3. Perform all multiplication and division as it occurs moving left to right in the
problem.
4. Perform all addition and subtraction as it occurs moving left to right in the
problem.
Here is a mnemonic device that can help you remember the order of operations:
After reading the order of operations a few times and having them memorized please try the non-graded practice quiz to see if you have them memorized in order.
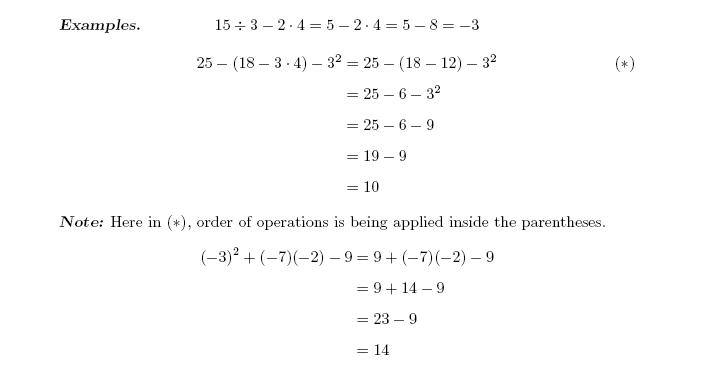
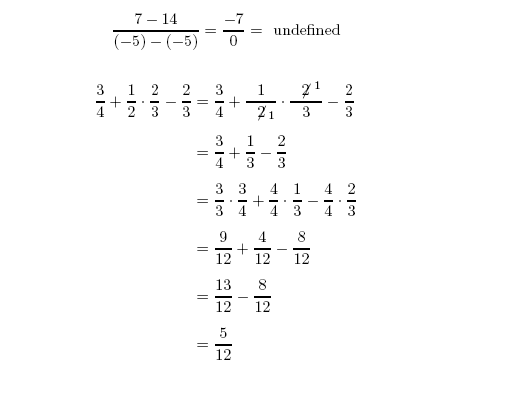
INSERT EXAMPLES ABOVE AS CAMTASIA MOVIES
Practice identifying which property of real numbers is demonstrated in the Lesson 2 Property Identification Practice Quiz ( This link will open in a new window)
Combine like terms to completely simplify each of the following:
7. 8x + 3x − 5x
8. 6x + 7y − 8x + 9y
9. 9x − 3x + 6 − 9
INSERT BUTTON- CLICK TO SEE ANSWERS
Remove the grouping symbols and combine like terms to completely simplify the following:
10. (8x − 3) − (2x + 5)
11. (7x + 5) − (4x − 6)
12. −(3x − 2) + (8x − 7)
13. (5x + 2y − 7) − (3x − 2y − 9)
INSERT BUTTON- CLICK TO SEE ANSWERS
Use order of operations to simplify each of the following:
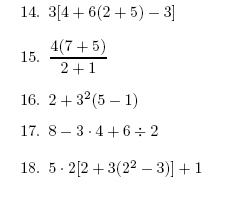
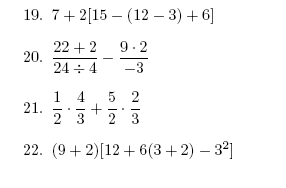
INSERT BUTTON - CLICK TO SEE ANSWERS
Please complete these problems with your Fly Pen and Fly Paper. Then submit your work to the lesson 2 homework drop box.
For instructions on the Fly Pen and Fly Paper please see the homework submission help. NEED TO LINK TO HELP PAGE